Answer:
a) charges of the opposite, b) Δq = q₄₂-q₄₁, force is repulsive
c) q = 5.27 10⁻⁵ C
Step-by-step explanation:
a) In electrostatic studies it is found that charges of the same sign repel and charges of the opposite sign attract.
On the other hand, as the force between the spheres is attractive, the charges on them are of different sign
b) When the balls touch, the charges are quickly distributed between the two spheres, therefore there are two possibilities:
* if the charges were equal and as they are of the opposite sign, they are neutralized, therefore the spheres remain uncharged
The force between them is zero
* if the charges are different, a residual charge remains
Δq = q₄₂-q₄₁
that is distributed between the two spheres and the force between them is repulsive
c) For this case the charges on the two spheres is equal
q₄₁ = q₄₂ = q
the force is repulsive so the charges are of the same sign.
We can apply Coulomb's law
F =

in this case
F =
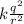
q =
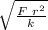
let's calculate
q =
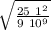 =
=
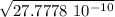
q = 5.27 10⁻⁵ C