Question

A toy rocket is launched with an initial velocity of 12.0 m/s in the horizontal direction from the roof of a 30.0-m-tall building. The rocket’s engine produces a horizontal acceleration of \left(1.60 m / s^{3}\right) t, in the same direction as the initial velocity, but in the vertical direction the acceleration is g , down-ward. Ignore air resistance. What horizontal distance does the rocket travel before reaching the ground?

Answer 1

The motion in the y direction.

The time taken by the toy rocket to hit the ground,

`$S=ut+(1)/(2)at^2$`

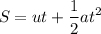
S = distance travelled = 30 m

u = 0 m/s

a =
`$9.8 \ m/sec^2$`

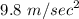
t= time in seconds

Therefore,
`$30 =(1)/(2)9.8 t^2$`

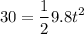
t = 2.47 sec

Now motion in the x direction,

u = 12 m/sec

`$a=1.6 t \ m/sec^3$`

Upon integration 'v' with respect to 't'

`$v=(1.6t^2)/(2)+12$`

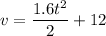
Once again integrating with respect to t,

`$s=(1.6t^3)/(6)+12 t$`

`$s=(1.6(2.47)^3)/(6)+12 (2.47)$`

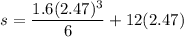
= 0.0176+29.64

= 29.65 m

Therefore, the toy rocket will hit the ground at 29.65 m from the building.