Answer:
193 mg
Explanation:
Exponential decay formula:
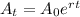
- where Aₜ = mass at time t, A₀ = mass at time 0, r = decay constant (rate), t = time
Our known variables are:
- 1998 to the year 2004 is a total of t = 6 years.
- The sample of radioactive isotope has an initial mass of A₀ = 360 mg at time 0 and a mass of Aₜ = 270 mg at time t.
Let's solve for the decay constant of this sample.
Using our new variables, we can now solve for Aₜ at t = 7 years, since we go from 2004 to 2011.
Our new initial mass is A₀ = 270 mg. We solved for the decay constant, r = 0.04794701.
The expected mass of the sample in the year 2011 would be 193 mg.