1 Answer

Lfbet · Answer 1 · 2022-10-25T18:49:21+0000

Answer:

The straight-line distance between the points is approximately 8.6.

Explanation:

The straight-line distance (
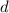) between the points is determined by the following Pythagorean identity:

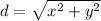$d = \sqrt{x^(2)+y^(2)}$ (1)

Where:

- Horizontal distance between the points.

- Vertical distance between the points.

Let consider that each square has a distance of 1 unit. If we know that
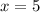x = 5 and
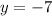y = -7 , then the straight-line distance is:

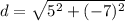
$d = \sqrt{5^(2)+(-7)^(2)}$

$d \approx 8.6$

The straight-line distance between the points is approximately 8.6.

Please log in or register to add a comment.