Question

population of a small town of $10,800 2002 the population has decreased at a rate of 2.5% each year write an exponential function to model the situation then find the population of the town of 2020​

Answer 1

The population of the town of 2020 is approximately 6847

Explanation:

The given population of the small town is 2002, P₀ = 10,800

The annual percentage decrease in the population, r = 2.5%

The date at which the new population,'P', is required = 2020

Therefore;

The number of years over which the population changes, 't', is given as follows;

t = 2020 - 2002 = 18

The number of years over which the population changes, t = 18 years

Given that the population is decreasing, we get;

The general formula for exponential decay is given as follows;

`P = P_0 \cdot (1 - r)^t`

Therefore, the population, 'P', of the town of 2020 which is in t = 18 years, is given as follows;

`P = 10,800 *(1 - 0.025)^(18) = 6847.10263915`

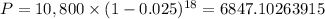
Given that the population is given in whole numbers, we round down to the nearest whole number population of people as follows;

The population of the town of 2020, P ≈ 6847.