Answer:
146.96
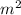
Explanation:
1. Approach
Divide this figure into two smaller shapes. Calculate the area of these smaller shapes, and then add the results. One can divide this shape into a semi-circle, and a triangle.
2. Area of the triangle
The formula to find the area of a triangle is;
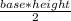
It is given that the base of the triangle is (14m) and the height is (10m). Substitute in these values, and solve for the area,
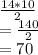
2. Area of the semi-circle
The area of the semi-circle can be found by finding the area of the larger circle, and then divide it by 2, since a semi-circle is half of a circle. The formula for the area of a semi-circle is;
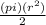
Where (pi) is the constant (3.1415...), (r) represents the radius of the circle, which by definition is half of the diameter. Substitute in the values and solve;

3. Find the total area;
Now add up the two values for the area of the figures;
Triangle + Semi-circle
= 70 + 76.96
= 146.96