Answer:
The particle traveled 4 inches over the interval.
Explanation:
What distance does the particle travel over the interval 0 ≤ t ≤ 1?
The distance is the integral of the velocity.
So
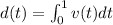
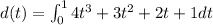
We have that:

So
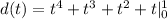
Which is:
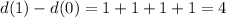
The particle traveled 4 inches over the interval.