Answer:
Surface Area = 384 m²
Explanation:
The given figure is a triangular prism.
The surface area of a triangular prism is made up of:
- 2 congruent triangles (the bases of the prism).
- 3 rectangles.
From inspection of the diagram, the dimensions of the triangular bases are:
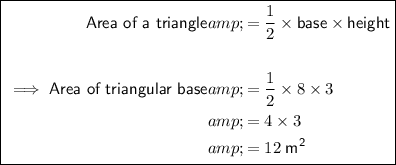
From inspection of the diagram, there are two congruent rectangles with dimensions:
and one rectangle with dimensions:
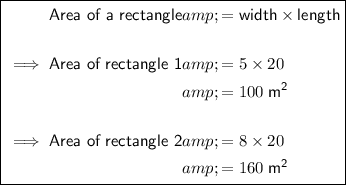
Therefore, the total surface area of the given triangular prism is:
