Question

HELPPP PLS!!!!Which of the following is equivalent to sum from n equals 1 to infinity of 10 times quantity negative seven eighths end quantity to the power of n question mark

Answer 1

-14/3

Explanation:

this is a geometric series with r = -7/8

The infinite sum of geometric series with |r| < 1 is

a1/(1-r)

We already have r, but we need to find a1 which is the first term of the series.

To find it, only plug in 1 for n, 10(-7/8) and this = -35/4

Now plug in r and a1 in the formula above:

`(-35/4)/(1+7/8)`

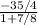
after simplifying it, you will get the infinite sum = -14/3