Question

Use the given conditions to write an equation for the line in the indicated

form. Show all work and write the solution on the written portion.
Passing through (5, 2) and parallel to the line whose equation is 9x+y-5=0;
A. point slope form; B.general form. __

Answer 1

let me assume that the general form is meant to be the standard form, that said

standard form for a linear equation means

• all coefficients must be integers, no fractions

• only the constant on the right-hand-side

• all variables on the left-hand-side, sorted

• "x" must not have a negative coefficient

now, keeping in mind that parallel lines have exactly the same slope, let's check for the slope of the equation above

`9x+y-5=0\implies y=\stackrel{\stackrel{m}{\downarrow }}{-9} x+5\qquad \impliedby \qquad \begin{array}ll \cline{1-1} slope-intercept~form\\ \cline{1-1} \\ y=\underset{y-intercept}{\stackrel{slope\qquad }{\stackrel{\downarrow }{m}x+\underset{\uparrow }{b}}} \\\\ \cline{1-1} \end{array}`

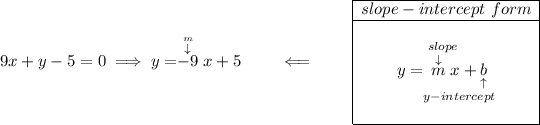
so we're really looking for the equation of a line whose slope is -9 and that it passes through (5 , 2)

`(\stackrel{x_1}{5}~,~\stackrel{y_1}{2})\hspace{10em} \stackrel{slope}{m} ~=~ - 9 \\\\\\ \begin{array}c \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies {\Large \begin{array}{llll} y-\stackrel{y_1}{2}=\stackrel{m}{- 9}(x-\stackrel{x_1}{5}) \end{array}} \\\\\\ y-2=-9x+45\implies y=-9x+47\implies {\Large \begin{array}{llll} \stackrel{standard~form}{9x+y=47} \end{array}}`