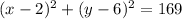Answer:
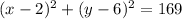
Explanation:

Given:
- Center = (2, 6)
- Point on the circle = (-3, 18)
Substitute the given center and point into the equation of a circle formula and solve for r²:

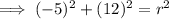
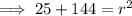
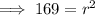

Therefore, the standard form of the equation of the circle with the given characteristics is: