Answer:
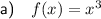
b) 4. horizontal shift of 4 units right
2. reflection in the x-axis
c) See attachment.
Explanation:
Part (a)
Given function:
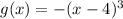
The parent function is:
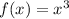
Part (b)
To transform the parent function f(x) to g(x):
1. Horizontal shift of 4 units right:
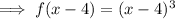
2. Reflection in the x-axis:
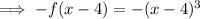
Part (c)
To sketch the graph of g(x):
- Sketch the graph of y = x³
- Translate it 4 units to the right.
- Reflect it in the x-axis.