Answer:
A could finish the whole work in 60 days
Explanation:
Both A and B can finish the work in 30 days.
That means both A and B can finish 1/30 part of the work in 1 day.
So in 20 days, both A and B can finish 20/30 = 2/3 part of the work.
Both A and B worked together for 20 days and completed 2/3 part of the work. Now 1/3 part of the work is remaining.
But after 20 days B left the work. Now A finished the remaining 1/3 part of the work alone.
Given A has completed the remaining 1/3 part of the work in 20 days. We are asked to find in how many days A could finish the whole work.
Let A could finish the whole work in x days.
That means A could finish 1/x part of the work in 1 day.
So in 20 days, A could finish 20(1/x) = 20/x part of the work.
But it's given that A finished the remaining 1/3 part of the work in 20 days.
Thus we are able to equate 20/x and 1/3.
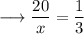
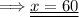
Hence A could finish the whole work in 60 days.