Question

NO LINKS!!

Find a logarithmic equation that relates y and x. (Round any numeric values to 3 decimal places.)

ln(y)=

x y
1 1.250
2 1.051
3 0.950
4 0.884
5 0.836
6 0.799

Answer 1

`\ln (y) = -0.250 \ln (x)+0.223`

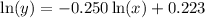
Explanation:

Given table:

`\begin{array}c\cline{1-2} \vphantom{\frac12}x & y\\\cline{1-2} \vphantom{\frac12} 1 & 1.250\\\cline{1-2} \vphantom{\frac12} 2 & 1.051\\\cline{1-2} \vphantom{\frac12} 3& 0.950\\\cline{1-2} \vphantom{\frac12} 4& 0.884\\\cline{1-2} \vphantom{\frac12} 5& 0.836\\\cline{1-2} \vphantom{\frac12} 6& 0.799\\\cline{1-2} \end{array}`

To convert y = axⁿ to linear form, take natural logs of both sides and rearrange:

`\begin{aligned}y=ax^n \implies \ln y &= \ln ax^n\\\implies \ln y &= \ln a + \ln x^n\\\implies \ln y &= \ln a + n \ln x\\\implies \ln y &=n \ln x+ \ln a\end{aligned}`

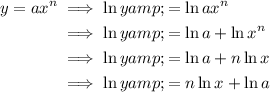
This is in the straight-line form y = mx + c.

Substitute the first values of x and y from the table into the natural log formula and solve for ln(a):

`\implies \ln 1.250= n \ln 1+\ln a`

`\implies \ln 1.250= n (0)+\ln a`

`\implies \ln a = \ln 1.250`

`\implies \ln a = 0.223\; \sf (3 \; d.p.)`

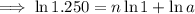
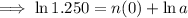
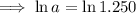
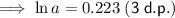
Substitute the found value of ln(a) and the last values of x and y from the table into the natural log formula and solve for n:

`\implies \ln 0.799= n \ln 6+\ln 1.250`

`\implies n \ln 6=\ln 0.799-\ln 1.250`

`\implies n =(\ln 0.799-\ln 1.250)/(\ln 6)`

`\implies n =-0.250\;\sf (3 \; d.p.)`

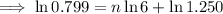
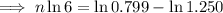
Substitute the found values of n and ln(a) into the formula to create an equation for ln(y):

`\boxed{\ln (y) = -0.250 \ln (x)+0.223}`