Answer:
Approximately
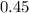 (assuming that the surface is level, the mass is moving at constant velocity, and that
(assuming that the surface is level, the mass is moving at constant velocity, and that
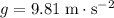 .)
.)
Step-by-step explanation:
With a power of
 , the work that would be done over
, the work that would be done over
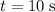 would be:
would be:
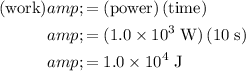 .
.
Divide work by distance to find the force that did the work:
 .
.
If this mass is moving at a constant velocity, the magnitude of friction on this mass will be equal to that of the external force,
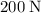 .
.
If the surface is level, the magnitude of the normal force on this mass will be equal to that of weight:
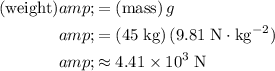 .
.
Divide the magnitude of friction by normal force to find the coefficient of friction:
 .
.