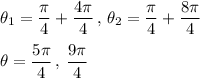Answer:
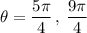
Explanation:
Given the equation
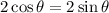 . We have to divide both sides by 2 which gives us
. We have to divide both sides by 2 which gives us
 . Then divide both sides by
. Then divide both sides by
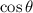 which gives us
which gives us
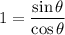 .
.
We know that:
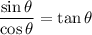
So we can rewrite the equation as
 .
.
We know that
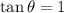 when
when
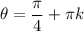 for
for
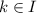 (k is any integer). However, the equation is given with the interval
(k is any integer). However, the equation is given with the interval
 . Therefore, we have to substitute k-values that satisfy the interval.
. Therefore, we have to substitute k-values that satisfy the interval.
It appears that only k = 1, 2 which gives us
 and
and
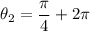 can be used since both satisfies both values and interval.
can be used since both satisfies both values and interval.
Simplifying both solutions: