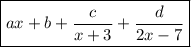Answer:
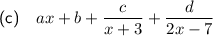
Explanation:
You want to know the form of the quotient and partial-fraction expansion of ...

Quotient
The degree of the numerator is 3, which is 1 more than the degree of the denominator. That means the quotient will an expression of degree 1, so will have the form ...
ax +b . . . . . . (eliminates choices A and B)
Remainder
The quadratic denominator can be factored as (x +3)(2x -7). These factors will be the denominators of the partial fractions. The numerator will be of lower degree, so will be a constant. That means the partial fractions will have the form ...
c/(x +3) + d/(2x -7) . . . . . . . (eliminates choice D)
The numerator of the remainder from polynomial division will always be of lower degree than the denominator of the fraction representing it.
The form of the quotient and remainder is ...