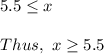Question

4x+6y=<4 2x+y=8 I need help with this equation

Answer 1

Answer: x≥5.5 y≤-3

Explanation:

`\displaystyle\\\left \{ {{4x+6y\leq 4\ \ \ \ (1)} \atop {2x+y=8\ \ \ \ \ (2)}} \right\\\\\\`

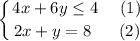
Divide both parts of the equation (1) by 2:

`\displaystyle\\\left \{ {{2x+3y\leq 2} \atop {2x+y=8}} \right\\\\\\`

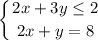
1)

`\displaystyle\\\left \{ {{2x+3y\leq 2\ \ \ \ (3)} \atop {2x=8-y\ \ \ \ \ (4)}} \right\\\\\\`

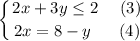
Let us substitute the value of 2x into equation (3):

`8-y+3y\leq 2\\\\8+2y\leq 2\\\\8+2y-8\leq 2-8\\\\2y\leq -6`

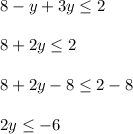
Divide both parts of the equation by 2:

`y\leq -3`

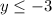
2)

`\displaystyle\\\left \{ {{2x+3y\leq 2\ \ \ \ (3)} \atop {y=8-2x\ \ \ \ \ (5)}} \right\\\\\\`

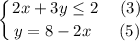
Let us substitute the value of y into equation (3):

`2x+3(8-2x)\leq 2\\\\2x+24-6x\leq 2\\\\24-4x\leq 2\\\\24-4x+4x\leq 2+4x\\\\24\leq 2+4x\\\\24-2\leq 2+4x-2\\\\22\leq 4x`

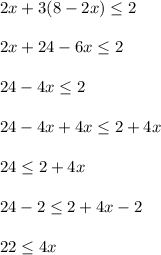
Divide both parts of the equation by 4:

`5.5\leq x\\\\Thus,\ x\geq 5.5`