Answer:

Explanation:
There are 3 total students in the club, so there are 3 possible first students that could be selected. After the first student is selected, there are 2 remaining students to choose from.
Since there are
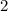 boys and
boys and
 girl in the club, the probability that a boy is selected as the first student is
girl in the club, the probability that a boy is selected as the first student is
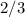 . The probability that a girl is selected as the second student, given that a boy was selected as the first student, is
. The probability that a girl is selected as the second student, given that a boy was selected as the first student, is
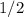 . Therefore, the probability of selecting a boy and a girl in that order is
. Therefore, the probability of selecting a boy and a girl in that order is
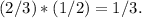
The same is true if the order is reversed, so the probability of selecting a girl and a boy is also
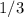 . Thus, the probability of selecting a boy and a girl in any order is
. Thus, the probability of selecting a boy and a girl in any order is
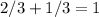 .
.
In summary, the probability of selecting a boy and a girl at random from the club is
 .
.