Answer:
1)
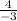
2)
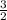
Explanation:
To find the slope, we can use the slope formula:
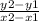
1. Through (4,-3) and (-2,5):
Slope =
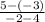
Slope =
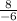
Simplified to:
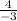
2. Through (2, -1) and (0, -4)
Slope =
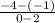
Slope =
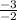
Slope =
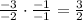
Meaning that the slope of 1) is
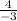 and the slope of 2) is
and the slope of 2) is
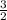 .
.
Hope this helps!