Question

The condenser transfers energy from the coolant to the air in the building.

When the total energy input to the heat pump system is 1560 kJ the temperature of the air
in the building increases from 11.6 °C to 22.1 °C.
The efficiency of the heat pump system is 87.5%.
The mass of the air inside the building is 125 kg.
Calculate the specific heat capacity of the air in the building.
Give your answer in standard form.

Answer 1

Approximately
`1.04 \; {\rm J \cdot g^(-1) \cdot K^(-1)}`.

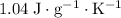Step-by-step explanation:

Multiply energy input by efficiency to find the useful energy output:

`\begin{aligned}& (\text{useful out}\text{put}) \\ &= (\text{efficiency})\, (\text{energy in}\text{put}) \\ &= (87.5\%)\, (1560\; {\rm kJ}) \\&= (0.875)\, (1560\; {\rm kJ}) \\ &= 1365\; {\rm kJ}\end{aligned}`.

In other words,
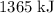`1365\; {\rm kJ}` of energy was supplied to the air in the building.

The standard unit of energy is Joules (
`{\rm J}`.) Apply unit conversion:

`\begin{aligned}(1365\; {\rm kJ})\, \left(\frac{10000\; {\rm J}}{1\; {\rm kJ}}\right) = 1.365* 10^(6)\; {\rm J}\end{aligned}`.

Let
`c` denote the specific heat capacity of the air in this building. Let
`m` denote the mass of the air.

Let
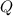`Q` denote the energy supplied to the air. Let
`\Delta T` denote the change in temperature. The equation
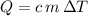`Q = c\, m\, \Delta T` relates these quantities.

In this question, the change in the temperature of the air in this building is:

`\Delta T = (22.1\; {\rm ^(\circ) C}) - (11.6\; {\rm ^(\circ) C}) = 10.5\; {\rm K}`.

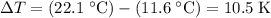Rearrange the equation
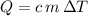`Q = c\, m\, \Delta T` to find specific heat capacity
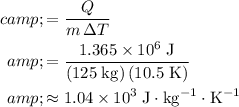`c`:

`\begin{aligned}c &= (Q)/(m\, \Delta T) \\ &= \frac{1.365 * 10^(6)\; {\rm J}}{(125\; {\rm kg})\, (10.5\; {\rm K})} \\ &\approx 1.04 * 10^(3)\; {\rm J \cdot kg^(-1) \cdot K^(-1)}\end{aligned}`.