Answer:
$9,712.12 (nearest cent)
Explanation:
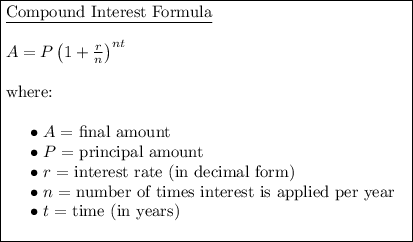
Given:
- P = $9,544
- r = 5.25% = 0.0525
- n = 12 (monthly)
- t = 4 months = 1/3 year
Substitute the given values into the formula and solve for A:
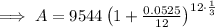
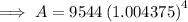
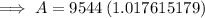
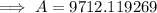
The balance of the account at the end of August will be $9,712.12 (nearest cent).