Question

F(x) = 2x³x² - 29x

What is the greatest possible number of turning points?

Answer 1

4

Explanation:

First we need to get some definitions out of the way

Definition of a turning point

• A turning point is a point of the graph where the graph changes from increasing to decreasing (rising to falling) or decreasing to increasing (falling to rising).

• A polynomial of degree n will have at most n−1 turning points.

Definition of degree of polynomial

• The degree of a polynomial is the degree of the term having the highest degree.

• The given polynomial is
  
  `f(x) = 2x^3x^2-29x`

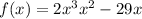
• The highest degree of
  `x` is the degree of the polynomial
  
• We know that when we multiply same terms with different exponents, the exponents get added. Therefore
  
  `x^3x^2 = x^(3 + 2) = x^5`

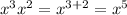
• So the polynomial can be re-written as:
  
  `2x^5 -29x`

• The highest degree of
  `x` is
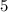  `5` and this is the degree of the polynomial
  
• So the maximum number of turning points
  `= 5-1 = 4`