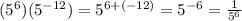Answer:
A.)
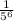
Explanation:
To simplify
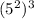 , use the exponent rule that states
, use the exponent rule that states
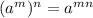
So,
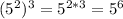
Use the same exponent rule to begin simplifying
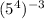 :
:
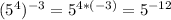
To further simplify
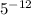 , use the exponent rule that states
, use the exponent rule that states
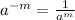
So,
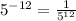
Then multiply the two new expressions as indicated in the original problem:
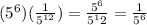
Alternatively, you could use the exponent rule that states
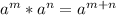
where
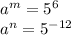
So,