Question

5.

The population of arctic foxes in Yukon can be modelled by the following equation:
P = 400 cos(30t - 180) + 1100
Where 't' represents the number of years since 1992.
a.
What was the population of arctic foxes in Yukon in 1992?

b. What are the maximum and minimum number of arctic foxes?

C. Sketch a graph that represents how the population of artic foxes changes over time (since 1992).

D. What will be the population of arctic foxes in 2030?

E. When was the first time the population of arctic foxes reached 1300 (since 1992)?

Answer 1

Explanation:

`P(t)=400cos(30t-180)+1100`

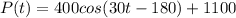
a. What was the population of arctic foxes in Yukon in 1992?

t' represents the number of years since 1992. So plugging in 0 gives the population in 1992.

`P(0)=400cos(30*0-180)+1100`

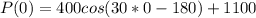
Subtract 180 from 0.

`P(0)=400cos(-180)+1100`

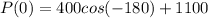
Apply the reference angle by finding the angle with equivalent trig values in the first quadrant. Make the expression negative because cosine is negative in the second quadrant.

`P(0)=400(-cos(0))+1100`

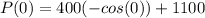
The exact value of cos (0) is 1.

`P(0)=400(-1*1)+1100`

`P(0)=400*-1+1100`

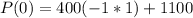
Multiply 400 by −1. Then add −400 and 1100.

`P(0)=700`

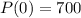
D. What will be the population of arctic foxes in 2030?

`P(38)=400cos(30(38)-180)+1100`

`P(38)=900`