Answer:
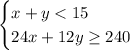
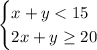
Explanation:
Part-time job
- Let x be the number of hours worked.
- $24 = pay per hour.
Internship
- Let y be the number of hours worked.
- $12 = pay per hour.
The student needs to work less than 15 hours a week:
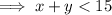
The student needs to earn at least $240 a week:
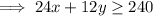
Therefore, the system of inequalities is:
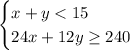
The second inequality can be simplified by dividing each term by 12:
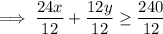
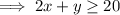
Therefore, a second system of inequalities is:
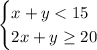
To solve the system of equalities, graph both inequalities and shade the overlapping region.
Any (x, y) point in the overlapping region is a solution to the system of inequalities.