Question

An aerosol spray can with a volume of 325 mL contains 3.00 g of propane (C3H8) as the propellant. What is the pressure, in atm, of the gas in the can at 28°C?

a) 5.17 atm
b) 228 atm
c) 0.00517 atm
d) 0.481 atm
e) 4.69 atm

Answer 1

A.) 5.17 atm

Step-by-step explanation:

According to the Ideal Gas Law, PV=nRT where:

P= pressure in atm

V= volume in L

n= moles of gas

R= ideal gas constant

T= temperature in K

To calculate moles of propane, first calculate the molecular weight:

`C=12.01(g)/(mol)\\H=1.01(g)/(mol)\\\\3(12.01(g)/(mol))+8(1.01(g)/(mol))\\36.03(g)/(mol)+8.08(g)/(mol)\\43.11(g)/(mol)`

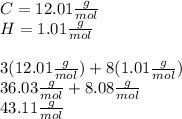
Then use that molecular weight to convert g to mol:

`3.0gC_3H_8((1mol)/(43.11g))=0.0696molC_3H_8`

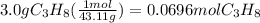
So, for this problem, let:

V=0.325 L

n=0.0696 mol

R=0.08205
`(L*atm)/(mol*K)`

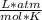
T=(28+273)K=301K

Next, rewrite the Ideal Gas Law to solve for P:

`P=(nRT)/(V)\\P=((0.0696mol)(0.08205(L*atm)/(mol*K))(301K))/(0.325L)\\P=5.29atm`

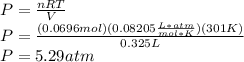
The result isn't exactly any of the answers, but I assume there was a difference in rounding somewhere. The only answer that is even remotely close is the 5.17 atm.