Question

Graph the quadratic
x^2+x-6=0

Answer 1

See attachment.

Explanation:

Given quadratic equation:

`x^2+x-6=0`

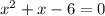
Therefore, the quadratic function is:

`y = x^2+x-6`

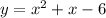
The graph of a quadratic function is a parabola.

• If the leading coefficient is positive, the parabola opens upwards.
• If the leading coefficient is negative, the parabola opens downwards.

Therefore, as the leading coefficient of the given quadratic function is positive, the parabola will open upwards.

x-intercepts

The x-intercepts are the points at which the curve crosses the x-axis, so when y = 0.

Therefore, to find the x-intercepts, factor the equation and solve for x:

`\implies x^2+x-6=0`

`\implies x^2+3x-2x-6=0`

`\implies x(x+3)-2(x+3)=0`

`\implies (x-2)(x+3)=0`

`\implies x-2=0 \implies x=2`

`\implies x+3=0 \implies x=-3`

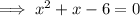
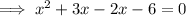
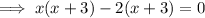
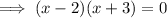
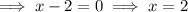
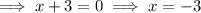
Therefore, the x-intercepts of the function are (2, 0) and (-3, 0).

Axis of symmetry

The axis of symmetry is the midpoint of the x-intercepts:

`\textsf{Midpoint}=(2+(-3))/(2)=(-1)/(2)=-0.5`

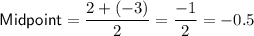
Therefore, the axis of symmetry of the function is x = -0.5.

Vertex

The axis of symmetry is the x-value of the vertex.

To find the y-value of the vertex, substitute the x-value into the function:

`x=-0.5 \implies (-0.5)^2+(-0.5)-6=-6.25`

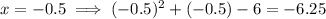
Therefore, the vertex of the function is (-0.5, -6.25).

y-intercept

The y-intercept is the point at which the curve crosses the y-axis, so when x = 0.

To find the y-intercept, substitute x = 0 into the function:

`x=0 \implies 0^2+0-6=-6`

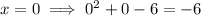
Therefore, the y-intercept of the function is (0, -6).

Graphing the function

To graph the function:

• Plot the vertex (-0.5, -6.25)
• Plot the x-intercepts (2, 0) and (-3, 0)
• Draw the axis of symmetry at x = -0.5
• Plot the y-intercept at (0, -6)
• Draw a curve symmetric about the axis of symmetry passing through the plotted points (upwards opening parabola).