Question

The following graph shows a seventh-degree polynomial:

Part 1: List the polynomial’s zeroes with possible multiplicities.
Part 2: Write a possible factored form of the seventh degree function.

Answer 1

Part 1:

-5, a multiplicity of 2

-1, a multiplicity of 1

4, a multiplicity of 3

7, a multiplicity of 1

Part 2:
`f(x)=(x+5)^2(x+1)(x-4)^3(x-7)`

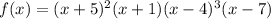
Explanation:

This question has to be done visually, and can is a little tricky, because depending on the way the graph intercepts the x-axis, there is going to be a different number of roots.

The roots are represented by the intersection of the function with the x-axis.

To start, take a look at the point
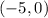`(-5,0)\\`, which intercepts the x-axis like a parabola(quadratic function). This means that -5 is a "double root" of the function, or it represents two roots of the function.

Now look at the point
`(-1,0)\\`, which intercepts the x-axis in a linear way, meaning that -1 is simply a "single-root" of the function, or it only represents one root.

So for the next root, look at
`(4,0)`, which intercepts the x-axis like a cubic function. This means that 4 is is a "triple-root" of the function, and represents 3 roots of the function.

And finally, we have one final interception at
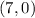`(7,0)\\`, and the interception is in a linear form, meaning 7 is simply a "single-root" to the function, or it only represents one root.

So we can conclude that the following are roots with their respective multiplicities

-5, a multiplicity of 2

-1, a multiplicity of 1

4, a multiplicity of 3

7, a multiplicity of 1

And totally, 2+1+3+1 gives us 7, which supports our answer because we know this is a seventh-degree polynomial.(1)

Now for Part 2

We simply take each root, and subtract it from x in our function, then apply our multiplicity as the exponent

So the function would be:
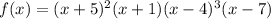`f(x)=(x+5)^2(x+1)(x-4)^3(x-7)`(2)

Hope this helps.