Question

1.Write the expression in expanded form. The subscript number can be write in

normal form. E.g. X1 + X1Y1, do not forget the correct parenthesis and the correct
order. *



2.For the equation in above evaluate the notation using the values below. Please
enter the exact value.
X1 =0
Y1 = 5
Z1 = 0
X2 = 1
Y2 = 26
Z2 = -2
X3 = 12
Y3 = -2
Z3 = 3
X4 = -1
Y4 = 25
Z4 = 24​

Answer 1

(a) Expanded form:

`((X_1 + X_1Y_1) - X_1Z_1) + ((X_2 + X_2Y_2) - X_2Z_2) + ((X_3 + X_3Y_3) - X_3Z_3) + ((X_4 + X_4Y_4) - X_4Z_4)`

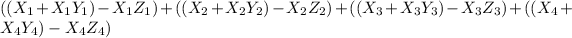
(b) The value of the expression: -21

Explanation:

Given

`\sum \limit^4_(i=1)\ ((X_i+X_iY_i) - X_iZ_i)`

Solving (a): The expanded form:

This means that we substitute the values of i from 1 to 4 in the above expression.

So, the expression becomes:

`((X_1 + X_1Y_1) - X_1Z_1) + ((X_2 + X_2Y_2) - X_2Z_2) + ((X_3 + X_3Y_3) - X_3Z_3) + ((X_4 + X_4Y_4) - X_4Z_4)`

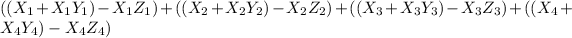
Solving (b): The value of the expression

To do this, we simply substitute the given values of X1, X2....... in the expression.

This gives:

So, the expression becomes:

`((0 + 0*5) - 0*0) + ((1 + 1*26) - 1*-2) + ((12 + 12*-2) - 12*3) + ((-1 + -1*25) - -1*24)`

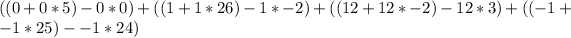
Simplify each bracket

`((0 + 0) - 0) + ((1 + 26) +2) + ((12 -24) - 36) + ((-1 -25) +24)`

`0 + 29 -48 -2`

`-21`

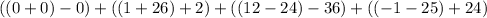
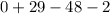
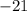
Hence, the result of the expression is -21