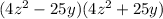Question

I need help with this by tonight pls or I will not get an updated grade it's hard for me to understand this ​

Answer 1

Find the Sum, Difference, or Product

1.
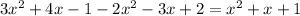
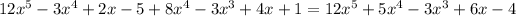
`3x^2 + 4x -1 - 2x^2 -3x + 2 = x^2 + x + 1`

2.
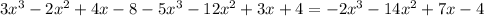
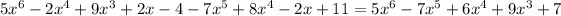
`12x^5 - 3x^4 + 2x -5+8x^4-3x^3+4x+1 = 12x^5 +5x^4-3x^3+6x-4`

3.
`3x^3 - 2x^2 + 4x-8-5x^3-12x^2+3x+4 = -2x^3-14x^2+7x-4`

4.
`5x^6 - 2x^4 +9x^3+2x-4-7x^5+8x^4-2x+11 = 5x^6-7x^5+6x^4+9x^3+7`

5.
`x^3+3x^2-10x-24` (Multiply the first two factor, then multiply the product to the last factor)

6.
`(7h+4)*(7h+4)= 49h^2+28h+28h+16 =49h^2+56h+16`

Factor the Sum or Difference of Cubes

Sum of Cubes Formula:
`a^3+b^3 = (a+b)(a^2-ab+b^2)\\`

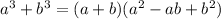
Difference of Cubes Formula:
`a^3-b^3 = (a-b)(a^2+ab+b^2)\\`

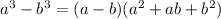
Just apply it to the equations.

1.
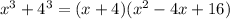
`x^3 + 4^3 = (x+4) (x^2 - 4x+16)`

2.
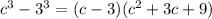
`c^3 - 3^3 = (c-3) (c^2+3c+9)`

3.
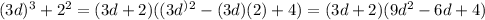
`(3d)^3 + 2^2 = (3d+2)((3d^)^2-(3d)(2)+4) = (3d+2)(9d^2-6d+4)`

4.
`5^3-(2b)^3= (5-2b)(5^2+(2b)(5)+(2b)^2) = (5-2b)(25+10b+4b^2)`

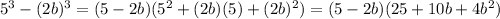
Factor the Polynomials Completely

Nt. I'm having a hard time with this one

1.
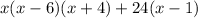
`x(x-6)(x+4)+24(x-1)`

2.
`2m^4 (m-4)(m-8)`

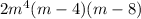
3.
`(6v+7)(3v+2)`

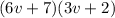
4.
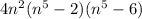
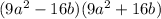
`4n^2(n^5-2)(n^5-6)`

5.
`(9a^2-16b)(9a^2+16b)`

6.
`(4z^2-25y)(4z^2+25y)`