Question

6x+6y=90 9x+2y=79 What pair of numbers solves the system?​

Answer 1

• (7, 8) is the required pair of numbers

---------------------------

Given system

• 6x + 6y = 90,
• 9x + 2y = 79.

Solve it by substitution

First, simplify the first equation by dividing all terms by 6:

• x + y = 15

Then solve it for y:

• y = 15 - x

Now, substitute this into the second equation:

• 9x + 2(15 - x) = 79
• 9x + 30 - 2x = 79
• 7x = 79 - 30
• 7x = 49
• x = 7

Finally, find the value of y:

• y = 15 - 7
• y = 8

The pair of numbers is (7, 8).

Answer 2

(7, 8)

Explanation:

equation 1:
`6x+6y=90`

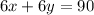
equation 2:
`9x+2y=79`

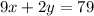
This system of equations can be solved for using the elimination method.

First, multiply both sides of the first equation by 3/2 to match the number of x's in both equations so that when we subtract the first equation from the second, the x's cancel and we can solve for y.

`\left((3)/(2)\right)\left(6x+6y\right)=(90)\left((3)/(2)\right)`

`9x + 9y = 135`

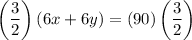
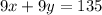
Next, subtract this from the second equation.

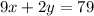`9x+2y=79` ← eq. 2

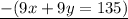`\underline{- (9x+9y=135)}` ← modified eq. 1

`0 - \, 7y \, = -56`

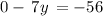
Then, solve for y by dividing by 7.

`7y = 56\\\overline{\, 7 \ } \ \ \ \ \overline{\ 7 \: }`

`y=8`

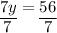
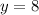
So, the y-coordinate of the solution pair is 8.

Now that we've solved for the y, all we have to do to get x is plug the solved y-value back into one of the equations. I'll use the first equation.

`6x+6y=90`

`6x + 6(8) = 90`

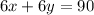
↓ multiplying out 6 and 8

`6x + 48 = 90`

↓ subtracting 48 from both sides

`6x = 90 - 48`

`6x = 42\\\overline{\: 6 \: } \ \ \ \ \, \overline{\: 6 \: }`

`x = 7`

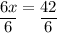
So, the x-coordinate of the solution pair is 7.

Finally, put the x- and y-coordinates together into an ordered pair in the form
`(x, y)`.

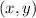(7, 8)