Question

Please help me. I know it’s 4 months but I don’t know what the total amount paid is including interest. If anyone could add the steps that would be awesome!

Answer 1

Divide by 12 to go from the APR to the monthly interest rate

(9.5%)/12 = 0.7916667%

The decimal form is 0.007916667 approximately.

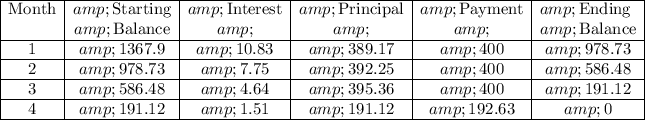
Multiply this with the starting balance $1367.90 and we get:

0.007916667*1367.90 = 10.8292 which rounds to 10.83

The interest payment for the 1st month is $10.83; which means the principal payment would be 400-10.83 = 389.17

This principal payment will then drop the balance from $1367.90 to 1367.90-389.17 = 978.73 dollars.

The value $978.73 is now the starting balance of the 2nd month. This process of...

• (a) Compute the interest
• (b) Subtract the interest from the $400 monthly payment to get the principal (with the exception of the last month as shown below)
• (c) Subtract the principal from the starting balance to get the ending balance.

is repeated until the balance is $0.

This is what the table would look like

`\begin{array}c \cline{1-6}\text{Month} & \text{Starting} & \text{Interest} & \text{Principal} & \text{Payment} & \text{Ending }\\& \text{Balance} & & & & \text{Balance}\\\cline{1-6}1 & 1367.9 & 10.83 & 389.17 & 400 & 978.73\\\cline{1-6}2 & 978.73 & 7.75 & 392.25 & 400 & 586.48\\\cline{1-6}3 & 586.48 & 4.64 & 395.36 & 400 & 191.12\\\cline{1-6}4 & 191.12 & 1.51 & 191.12 & 192.63 & 0\\\cline{1-6}\end{array}`

Many credit card companies and banks offer free calculators on their website, or you can find similar free calculators online elsewhere, that will quickly calculate a table like shown above. It is called an amortization table or amortization schedule.

With the exception of the 4th month, each row has the principal and interest add to the $400 monthly payment. The last row will have a payment of 1.51+191.12 = 192.63 dollars.

The total amount paid back would be 400+400+400+192.63 = 1392.63 dollars. This is the sum of the values in the "payment" column.

-------------------------------------------------------------------------

Here's another way to determine the answer.

The monthly payment formula is

`\displaystyle P = (Li)/(1 - (1+i)^(-n))`

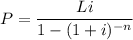
where,

• P = monthly payment
• L = loan amount, aka starting balance
• i = monthly interest rate in decimal form
• n = number of months

In this case,

• P = 400
• L = 1367.90
• i = 0.007916667 approximately (calculated earlier)
• n = unknown

Let's solve for the variable n. We'll need logarithms to isolate the exponent.

Part 1

`\displaystyle P = (Li)/(1 - (1+i)^(-n))\\\\\\\displaystyle 400 = (1367.90*0.007916667)/(1 - (1+0.007916667)^(-n))\\\\\\\displaystyle 400 = (10.8292087893)/(1 - (1.007916667)^(-n))\\\\\\\displaystyle 400(1 - (1.007916667)^(-n)) = 10.8292087893\\\\\\`

Part 2

`\displaystyle 1 - (1.007916667)^(-n) = 10.8292087893/400\\\\\\\displaystyle 1 - (1.007916667)^(-n) = 0.0270730\\\\\\\displaystyle -(1.007916667)^(-n) = 0.0270730-1\\\\\\\displaystyle -(1.007916667)^(-n) = -0.9729270\\\\\\\displaystyle (1.007916667)^(-n) = 0.9729270\\\\\\`

Part 3

`\displaystyle \log((1.007916667)^(-n)) = \log(0.9729270)\\\\\\\displaystyle -n*\log(1.007916667) = \log(0.9729270)\\\\\\\displaystyle n = -\log(0.9729270)/\log(1.007916667)\\\\\\\displaystyle n = 3.4805965\\\\\\`

Each decimal value is approximate.

That value of n then rounds up to the nearest integer n = 4

We do not round to n = 3 since that would be one month short, as shown in the table above.

You can use a graphing calculator to quickly solve that equation for the variable n. It can be used as a way to visually confirm the answer.