Question

Ok, my let’s try this again. can someone please help fill out the ? for this table.

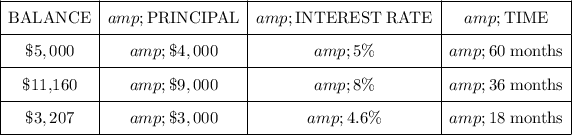
Answer 1

60 months

$9,000

4.6%

Explanation:

`\boxed{\begin{minipage}{6.5cm}\underline{Simple Interest Formula}\\\\$ A = P(1 + rt)$\\\\where:\\\\ \phantom{ww}$\bullet$ $A =$ final amount \\ \phantom{ww}$\bullet$ $P =$ principal amount \\ \phantom{ww}$\bullet$ $r =$ interest rate (in decimal form) \\ \phantom{ww}$\bullet$ $t =$ time (in years) \\ \end{minipage}}`

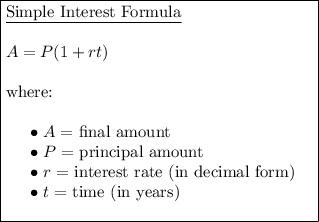
Given:

• A = $5,000
• P = $4,000
• r = 5% = 0.05

Substitute the given values into the formula and solve for t:

`\implies 5000=4000(1+0.05t)`

`\implies (5000)/(4000)=1+0.05t`

`\implies1.25=1+0.05t`

`\implies 0.05t=1.25-1`

`\implies 0.05t=0.25`

`\implies t=(0.25)/(0.05)`

`\implies t=5\; \rm years=60\; months`

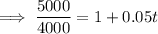
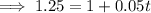
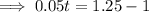
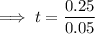
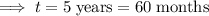
Given:

• A = $11,160
• r = 8% = 0.08
• t = 36 months = 3 years

Substitute the given values into the formula and solve for P:

`\implies 11160=P(1+0.08 \cdot 3)`

`\implies 11160=P(1.24)`

`\implies P=(11160)/(1.24)`

`\implies P=\$9000`

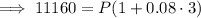
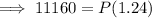
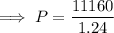
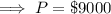
Given:

• A = $3,207
• P = $3,000
• t = 18 months = 1.5 years

Substitute the given values into the formula and solve for r:

`\implies 3207=3000(1+r \cdot 1.5)`

`\implies (3207)/(3000)=1+1.5r`

`\implies 1.069=1+1.5r`

`\implies 1.5r=1.069-1`

`\implies 1.5r=0.069`

`\implies r=(0.069)/(1.5)`

`\implies r=0.046`

`\implies r=4.6\%`

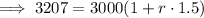
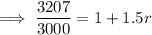
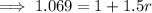
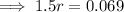
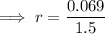
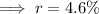
Therefore, the completed table is:

`\begin{array}c\cline{1-4} \vphantom{\frac12} \rm BALANCE & \rm PRINCIPAL & \rm INTEREST \; RATE & \rm TIME \\\cline{1-4} \vphantom{\frac12} \$5,000 & \$4,000 & 5\% & \rm 60 \; months\\\cline{1-4} \vphantom{\frac12} \$ 11,\!160 & \$9,000 & 8\% & \rm 36 \; months \\\cline{1-4} \vphantom{\frac12} \$3,207 & \$3,000 & 4.6\% & \rm 18 \; months\\\cline{1-4} \end{array}`