Question

planet a has twice the mass of planet b. from this information, what can we conclude about the escape speed for planet a compared to that of planet b?

Answer 1

Assume that the two planets have the same radius. The escape velocity of planet a(with twice the mass) will be
`√(2)` times the escape velocity of planet b.

Step-by-step explanation:

Let
`G` denote the gravitational constant.

Consider a spherical planet of mass
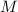`M` and radius
`r`. If an object of mass
`m` is on the surface of the planet, the gravitational potential energy
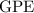`\text{GPE}` of that object will be:

`\displaystyle \text{GPE} = \left(-(G\, M\, m)/(r)\right)`.

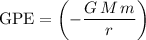If this object is moving at a speed of
`v`, the kinetic energy
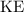`\text{KE}` of this object will be
`\text{KE} = (1/2)\, m\, v^(2)`.

If this object is moving at the escape velocity
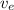`v_(e)` of the planet, the
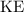`\text{KE}` of this object will be equal to the opposite
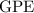`\text{GPE}`. In other words:

`\begin{aligned}(1)/(2)\, m\, {v_e}^(2) &= \text{KE} \\ &= (-\text{GPE}) \\ &= (G\, M\, m)/(r)\end{aligned}`.

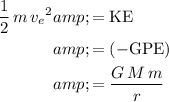Rearrange this equation to find escape velocity
`v_(e)`:

`\begin{aligned}{v_(e)}^(2) &= (2\, G\, M)/(r)\end{aligned}`.

`\begin{aligned}{v_(e)} &= \sqrt{(2\, G\, M)/(r)}\end{aligned}`.

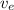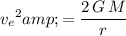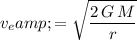Assume that the radius
`r` of the planet is constant. Based on this equation, escape velocity
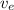`v_(e)` will be portional to
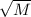`√(M)`, the square root of the mass of the planet.

Hence, if the radius of planet a and planet b are equal, the escape velocity of planet a will be
`√(2)` times the escape velocity of planet b.