Answer:
new pressure = 2.86 atm
Step-by-step explanation:
To solve the given problem, we have to use the 'combined gas law', which is expressed in the formula:
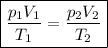 .
.
From the question, we know that the initial volume of the gas is 9.5 L, the initial pressure is 3.3 atm, and the initial temperature is 279 K. Therefore, V₁ = 9.5, p₁ = 3.3, and T₁ = 279.
We are also told that the gas temperature increases to 303 K and the volume increases to 11.9 L. Therefore, T₂ = 303 and V₂ = 11.9. We are then asked to calculate the new pressure (p₂).
To do this, we have to substitute the known values into the equation and solve it for p₂:

⇒
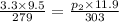
⇒

⇒
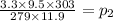
⇒

Therefore, the new pressure of the gas is 2.86 atm.