Question

Can a function of a value input be defined and it's limit around that value not exist?​

Answer 1

yes

Explanation:

You want to know if a function can have the characteristic that the limit at a point does not exist, but the function is defined at that point.

Undefined limit

A function has no limit at a point if the limit from the left is different from the limit approaching from the right.

Consider the sign function ...

`\text{sign}(x)=\begin{cases}-1,&x < 0\\0,&x=0\\1,&x > 0\end{cases}`

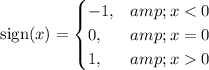
The left limit as x → 0 is -1; the right limit as x → 0 is +1, so the limit as x → 0 "does not exist." However, the function is defined at x=0.

The function is defined everywhere, but the limit as x→0 does not exist.