Question

3. A beam of light passes through the air (n=1.00) and enters a diamond (n=2.42) at an angle of incidence of 40 degrees. Use Snell’s Law to find the angle of refraction in the diamond. If the diamond is placed in a tank of water (n=1.33) and the beam of light enters the diamond at the same angle of incidence, what would be the new angle of refraction? Show all work.

Answer 1

(a) θ₂ = 15.4°

(b) θ₂ = 28.9°

Step-by-step explanation:

Snell's Law can be written as follows:

`n_1Sin\theta_1 = n_2Sin\theta_2`

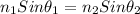
where,

n₁ = incident index

n₂ = refracted index

θ₁ = Angle of incidence

θ₂ = Angle of refraction

(a)

n₁ = incident index = 1

n₂ = refracted index = 2.42

θ₁ = Angle of incidence = 40°

θ₂ = Angle of refraction = ?

Therefore,

`(1)(Sin\ 40^o) = (2.42)Sin\theta_2\\\theta_(2) = Sin^(-1)(0.26561)`

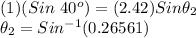
θ₂ = 15.4°

(b)

n₁ = incident index = 1

n₂ = refracted index = 1.33

θ₁ = Angle of incidence = 40°

θ₂ = Angle of refraction = ?

Therefore,

`(1)(Sin\ 40^o) = (1.33)Sin\theta_2\\\theta_(2) = Sin^(-1)(0.4832)`

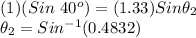
θ₂ = 28.9°