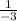Answer:
Explanation:
The given equation is not instandard form. First standard form is neccesary.
- Subtract 12y to both sides
The result is 3x + 108 = 12y which is equal to 12y = 3x + 108
The slope is represented in this form as m (Y = mx + b)
(with b as the initial value)
Therefore, 3 is the slope.
To find its perpendicular slope.
To find it's perpendicular, know that it is the negative reciprocal.
- First negate 3 to become -3 and find it's reciprocal
- To find the reciprocal, divide 1 by -3
FInally, the slope perpendicular to the line whose equation is 3x - 12y = -108 is