Question

Trigonometry, NEED HELP ASAP

Answer 1

`sin(\theta_1) = (√(357) )/(29)`

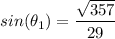
Explanation:

The parameters of the angle θ₁ are;

The location of θ₁ = Quadrant II

cos(θ₁) = -22/29

We note the following;

1) The sine of an angle in quadrant II is positive

2) The cosine of an angle in quadrant II is negative,

2) The cos of an angle = The adjacent leg length to the reference angle divided by the hypotenuse length of a right triangle

3) With regards to the right triangle for finding cos(θ₁)

The adjacent leg length = -22 (The x-axis is negative in quadrant II)

The hypotenuse length = 29

The negative sign is obtained from the value of cosine in the quadrant

Therefore, by Pythagoras' theorem, for a right triangle, we have;

The opposite leg length to 'θ₁' = √(29² - 22²) = √(357)

`sin\angle X = (Opposite \ leg \ length)/(Hypotenuse \ length)`

Therefore, we have;

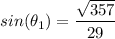`sin(\theta_1) = (√(357) )/(29)`.