Answer:
D. y = 2x - 2
Explanation:
1) First, find the slope of the given line. Place it in slope-intercept form to identify its slope easily. Isolate the y in the equation:

A line placed in slope-intercept form is represented by the formula
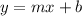 . The
. The
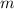 , or the coefficient of the x-term, represents the slope. Thus, the slope of this line is 2.
, or the coefficient of the x-term, represents the slope. Thus, the slope of this line is 2.
2) Lines that are parallel share the same slope. So, the slope of the parallel line will have 2 as its slope as well.
We now have enough information to write the equation of the line in point-slope form. From there, we can convert it to slope-intercept form and find out which option is correct.
Use the point-slope formula
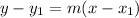 and substitute values for
and substitute values for
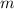 ,
,
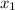 , and
, and
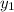 . Since
. Since
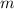 represents the slope of the line, substitute 2 in its place. Since
represents the slope of the line, substitute 2 in its place. Since
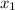 and
and
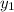 represent the x and y values of a point the line intersects, substitute the x and y values of (4,6) into the formula as well. Then, with the resulting equation, isolate y like before to find which option is correct:
represent the x and y values of a point the line intersects, substitute the x and y values of (4,6) into the formula as well. Then, with the resulting equation, isolate y like before to find which option is correct:
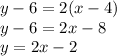
So, option D is correct.