Answer:
 to the east.
to the east.
Step-by-step explanation:
When an object of mass
 travels at a velocity of
travels at a velocity of
 , the momentum
, the momentum
 of that object will be
of that object will be
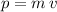 .
.
Let
 denote the mass of one ball.
denote the mass of one ball.
Before the collision:
- Momentum of the cue ball:
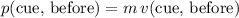 .
. - Momentum of the numbered ball:
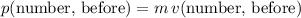 .
.
Hence, the total momentum before the collision was:
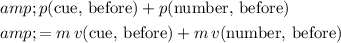 .
.
Likewise, the total momentum right after the collision would be:
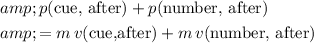 .
.
Momentum is supposed to be conserved during the collision. In other words, total momentum should be the same immediately before and after the collision. Hence:
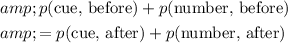 .
.
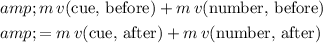 .
.
Assume that positive velocities denote motion to the east.
In this question,
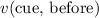 ,
,
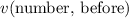 , and
, and
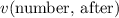 are all given. Rearrange this equation to find
are all given. Rearrange this equation to find
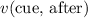 :
:
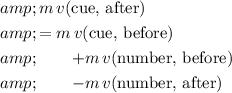 .
.
 .
.
Since the result is greater than zero, the direction of motion of the cue ball after the collision will also be to the east.