Answer:
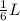 or 166.667 ml of water must be evaporated in order to make this solution 3M.
or 166.667 ml of water must be evaporated in order to make this solution 3M.
Step-by-step explanation:
1. Analyse the information.
When the problem states "250ml of 1M Ca(OH)₂", it means that you have 250ml of a solution that contains 1 mole of solute (Ca(OH)₂) for each liter of the solution.
In order to make a 1M solution be a 3M solution, we'll have to remove some of the solvent liquid, in order to make it more concentrated.
2. Express the molarity.
The original molarity is: M= Moles of solute (n) / Liters of solution (v).
3. Find the molarity.
Since the problem states that the original molarity is 1M, we have 1 mole of solute per liter of solution in the original solution. Therefore, initial molarity is:
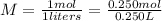
250ml= 0.250 L.
4. Convert the molarity to 3M.
As you may see, in order to make the molarity fraction equal 3M, we'll have to multiply the numerator by 3 or divide the denominator by the same number:
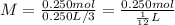
5. Calculate the amount of solution evaporated.
The amount of solution evaporated is given by the difference between the denominator of the molarity of the original expression and the new expression:
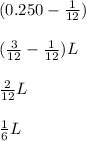
6. Conclude.
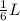 or 166.667 ml of water must be evaporated in order to make this solution 3M. The ending product will have the following molarity:
or 166.667 ml of water must be evaporated in order to make this solution 3M. The ending product will have the following molarity:
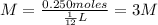 .
.