Addition of fractions:
Answer:
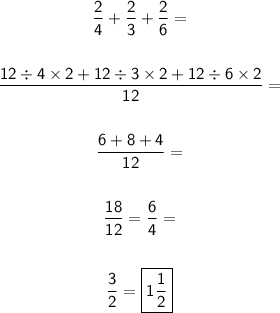
How to add heterogeneous fractions:
Heterogeneous fractions are fractions that have different denominators.
To add heterogeneous fractions, follow these steps:
- Find the common denominator
- This is the l.c.m of the denominators.
- Divide the common denominator by the original denominator and multiply by the numerator.
- Write the results obtained in the previous step as numerators with the sign of the sum between them.
- The denominator is the common denominator.
- Add the numerators and keep the same denominator.
- Simplify the result if possible.