Question

Pls help! picture is below. pls help by pst sunday december 4 , 2022 12:00 p.m.

Answer 1

Answer: August 12th

Step-by-step explanation: 4 and 6 have common multiples of 2, so you need to multiply 6 by 2 and 4 by 3. Both get you 12, so the earliest they have tennis practice is the 12th of August (12 days after July 31st).

Answer 2

August 12

Explanation:

To solve this question, we need to find the Least Common Multiple (LCM) of 4 and 6.

Since we know that Nick and Mark both had practice on July 31st, we can simply add {LCM of 4 and 6} days to that date to get when they will next both have practice.

To find the LCM of 4 and 6, find the prime factorization of both:

`4 = 2 * 2`
`6 = 2 * 3`

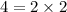
Now, take the largest quantity of each distinct prime number and multiply them together:

The largest number of 2's is 2. The largest number of 3's is 1. So, we multiply

`(2 * 2) * (1 * 3)`

`= 4 * 3`

`= 12`

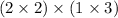
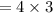
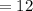
Therefore, the LCM of 4 and 6 is 12.

This means that Nick and Mark will have practice on the same day in 12 days.

The last step to solve this problem is to add 12 days to July 31.

`\textrm{July } 31 + 12 \textrm{ days} = \textrm{August } 12`

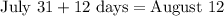
Nick and Mark will next have practice on the same day on August 12.