Answer:
(-3, -7).
Explanation:
1. Write the expressions.
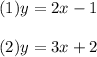
2. Write equation 1 and substitute its y value by the argument of expression 2.
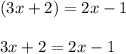
3. Subtract 2 in both sides of the equation.
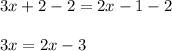
4. Subtract 2x from both sides of the equation.
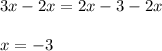
5. Verify the answer.
To see of the answer is correct, both of the equation should give the same y value for the x value of -3.
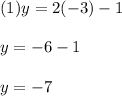
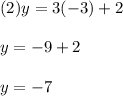
6. Express the final result.
(x, y)
(-3, -7).