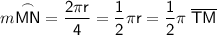Question

Please need help with this ASAP

Answer 1

`\sf (1)/(2) \pi \;\overline{TM}`

Explanation:

The angle at the circumference in a semicircle is a right angle.

Therefore, as
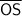`\sf \overline{OS}` is the diameter of circle T, and point Q is on the circumference of the circle:

• m∠SQO = 90°

Therefore, if m∠MTN = m∠SQO then:

• m∠MTN = 90°

Angles around a point sum to 360°.

As 90° is one quarter of 360°, the measure of arc MN is one quarter of the circumference of circle T.

The circumference of a circle is 2πr, where r is the radius.

Therefore:

`m\sf \overset{\frown}{MN}=(2 \pi r)/(4)=(1)/(2) \pi r=(1)/(2) \pi \;\overline{TM}`