Question

What is the equation of the line of symmetry for the parabola represented by the equation y=−2(x−7^)2+11?

Enter your answer as the correct equation, like this: x = 42

Answer 1

x = 7

Explanation:

`\boxed{\begin{minipage}{5.6 cm}\underline{Vertex form of a quadratic equation}\\\\$y=a(x-h)^2+k$\\\\where:\\ \phantom{ww}$\bullet$ $(h,k)$ is the vertex. \\ \phantom{ww}$\bullet$ $a$ is some constant.\\\end{minipage}}`

Given equation:

`y=-2(x-7)^2+11`

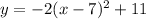
The given equation is in vertex form.

The axis of symmetry of a parabola with a vertical axis of symmetry is:

• x = h

where h is the x-value of the vertex.

As the vertex of the given equation is (7, 11), the axis of symmetry is:

• x = 7