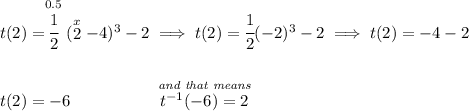Question

T(x)=.5(x-4)^3-2 , find t^-1(?)=2

Answer 1

well, we could just find the inverse of t(x) and then plug in the "2" and get it.

OR

we can just recall that "the inverse of t(x) has a domain that's the same as the range of t(x)"

what the heck all that means?

well, it means that for any (a , b) pair in t(x), the inverse t⁻¹(x) has an exact pair but sideways, namely (b , a).

so, if we want t⁻¹( x ) = 2, that means that t⁻¹( a ) has a pair of ( a , 2 ), well, hell, that also means that t(x) has a pair that ( 2 , a ).

so, if we want to know what "a" is, let's just find f(2), because since t⁻¹( a ) = 2, then t( 2 ) = a :)

`t(2)=\stackrel{0.5}{\cfrac{1}{2}}(\stackrel{x}{2}-4)^3 - 2\implies t(2)=\cfrac{1}{2}(-2)^3 -2\implies t(2)=-4-2 \\\\\\ t(2)=-6\hspace{5em}\stackrel{\textit{and that means}}{t^(-1)(-6)=2}`