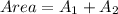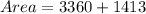Question

The design for the palladium window shown includes a semicircular shape at the top. The bottom is formed by squares of equal size. A shade for the window will extend 4 inches beyond the perimeter of the window, shown by the dashed line around the window. Each square in the window has an area of 169 in2. Round your answers to the nearest whole number.

Answer 1

`(a)\ Area = 3765.32`

`(b)\ Area = 4773`

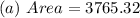
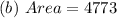
Explanation:

Given

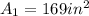`A_1 = 169in^2` --- area of each square

`Shade = 4in`

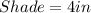
See attachment for window

Solving (a): Area of the window

First, we calculate the dimension of each square

Let the length be L;

So:

`L^2 = A_1`

`L^2 = 169`

`L = \sqrt{169`

`L=13`

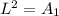
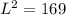
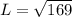
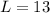
The length of two squares make up the radius of the semicircle.

So:

`r = 2 * L`

`r = 2*13`

`r = 26`

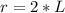
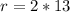
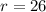
The window is made up of a larger square and a semi-circle

Next, calculate the area of the larger square.

16 small squares made up the larger square.

So, the area is:

`A_2 = 16 * 169`

`A_2 = 2704`

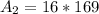
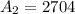
The area of the semicircle is:

`A_3 = (\pi r^2)/(2)`

`A_3 = (3.14 * 26^2)/(2)`

`A_3 = 1061.32`

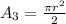
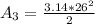
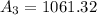
So, the area of the window is:

`Area = A_2 + A_3`

`Area = 2704 + 1061.32`

`Area = 3765.32`

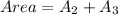
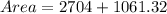
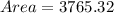
Solving (b): Area of the shade

The shade extends 4 inches beyond the window.

This means that;

The bottom length is now; Initial length + 8

And the height is: Initial height + 4

In (a), the length of each square is calculated as: 13in

4 squares make up the length and the height.

So, the new dimension is:

`Length = 4 * 13 + 8`

`Length = 60`

`Height = 4*13 + 4`

`Height = 56`

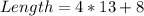
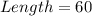
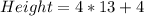
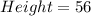
The area is:

`A_1 = 60 * 56 = 3360`

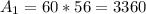
The radius of the semicircle becomes initial radius + 4

`r = 26 + 4 = 30`

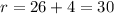
The area is:

`A_2 = (3.14 * 30^2)/(2) = 1413`

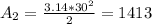
The area of the shade is:

`Area = A_1 + A_2`

`Area = 3360 + 1413`

`Area = 4773`